数据结构三之二叉搜索树的增删查

发表于 | 分类于

有了二叉树的基础, 我们继续学习二叉搜索树.

二叉搜索树的定义

二叉查找树(Binary Search Tree, 简称”BST”), 又名”二叉搜索树”或”二叉排序树”:
它或者是一棵空树，或者是具有下列性质的二叉树： 若它的左子树不空，则左子树上所有结点的值均小于它的根结点的值； 若它的右子树不空，则右子树上所有结点的值均大于它的根结点的值； 它的左、右子树也分别为二叉排序树。

. . .

注 :
我们本文中二叉树的二叉链式存储方案的代码表示为

1
2
3
4
5
6
typedef struct BinaryTreeNode
{
	void *data;
	BinaryTreeNode *LeftNode;
	BinaryTreeNode *RightNode;
}BTN, *BTNP;

二叉搜索树的查询

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
BTNP SearchBST(BTNP btnp, char key_data)
{
	while (btnp)
	{
		if ( key_data < (*(char *)(btnp->data)) )
		{
			btnp = btnp->LeftNode;
		}
		else if ( key_data >(*(char *)(btnp->data)) )
		{
			btnp = btnp->RightNode;
		}
		else
		{
			cout << "found" << endl;
			return btnp;
		}
	}
	cout << "not found" << endl;
	return nullptr;
}

二叉搜索树的插入

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
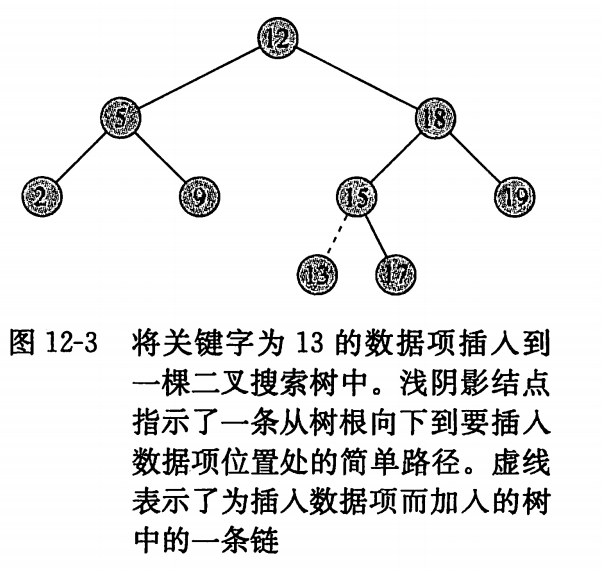
49
void InsertBST(BTNP &btnp, char key_data)
{

	char * temp_key_data = new char;
	*temp_key_data = key_data;

	BTNP new_btnp = new BTN;
	new_btnp->data = temp_key_data;
	new_btnp->LeftNode = nullptr;
	new_btnp->RightNode = nullptr;

	if (!btnp)
	{
		btnp = new_btnp;
		return;
	}

	BTNP temp_btnp = btnp;
	BTNP parent_btnp = nullptr;
	bool is_left = true;
	while (temp_btnp)
	{
		parent_btnp = temp_btnp;
		if (key_data < (*(char *)(temp_btnp->data)))
		{
			temp_btnp = temp_btnp->LeftNode;
			is_left = true;
		}
		else if (key_data > (*(char *)(temp_btnp->data)))
		{
			temp_btnp = temp_btnp->RightNode;
			is_left = false;
		}
		else
		{
			cout << "already has a same key_data" << endl;
			return;
		}
	}

	if (is_left == true)
	{
		parent_btnp->LeftNode = new_btnp;
	}
	else
	{
		parent_btnp->RightNode = new_btnp;
	}
}

二叉搜索树之删除某个结点

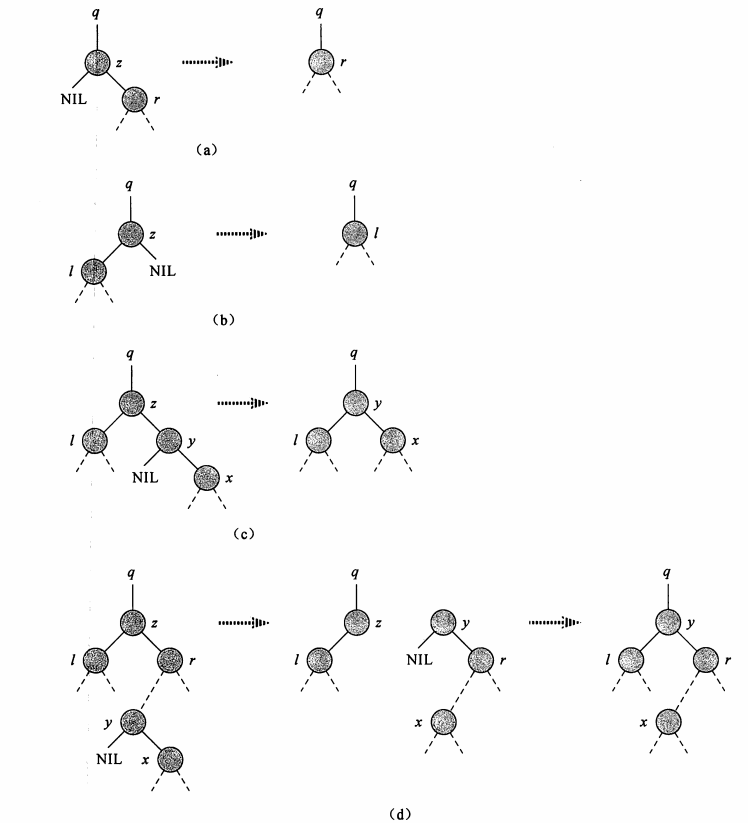
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
141
142
143
144
145
146
147
148
149
150
151
152
153
154
155
156
157
158
159
160
161
162
163
164
165
166
167
168
169
170
171
172
173

void DeleteBinaraySearchTree(BTNP &btnp, char key)
{
	// 第一步 : 查找是否有这个key
	BTNP parentBTNP = NULL;
	bool isLeft = true;

	BTNP tempBNTP = btnp;

	while (tempBNTP)
	{
		if (*(char*)tempBNTP->data > key)
		{
			parentBTNP = tempBNTP;
			tempBNTP = tempBNTP->LeftNode;
			isLeft = true;
		}
		else if (*(char*)tempBNTP->data < key)
		{
			parentBTNP = tempBNTP;
			tempBNTP = tempBNTP->RightNode;
			isLeft = false;
		}
		else
		{
			break;
		}
	}

	if (!tempBNTP)
	{
		cout << "not found this key!!" << endl;
		return;
	}

	cout << "found this key!!" << endl;

	// 第二步 : 我们得处里key结点没有父结点的情况
	if (!parentBTNP)
	{
		delete (char *)btnp->data;
		btnp->data = NULL;

		delete btnp;
		btnp = NULL;

		cout << "test" << endl;

		return;
	}
	else
	{
		// 第三步 : 我们得处里key结点有父结点的4种情况

		// 情况1
		if (tempBNTP->LeftNode == NULL && tempBNTP->RightNode == NULL)
		{
			if (isLeft)
			{
				parentBTNP->LeftNode = NULL;
			}
			else
			{
				parentBTNP->RightNode = NULL;
			}
		}
		// 情况2
		else if (tempBNTP->LeftNode == NULL)
		{
			if (isLeft)
			{
				parentBTNP->LeftNode = tempBNTP->RightNode;
			}
			else
			{
				parentBTNP->RightNode = tempBNTP->RightNode;
			}
		}
		// 情况3
		else if (tempBNTP->RightNode == NULL)
		{
			if (isLeft)
			{
				parentBTNP->LeftNode = tempBNTP->LeftNode;
			}
			else
			{
				parentBTNP->RightNode = tempBNTP->LeftNode;
			}
		}
		// 情况4
		else if (tempBNTP->LeftNode != NULL && tempBNTP->RightNode != NULL)
		{
			// 情况4比较复杂, 我们得找到key结点的后继
			// (后继 : 一个结点x的后继是大于x.key的最小关键字的结点)
			// 因为情况4中的key结点左孩子和右孩子都不为空, 
			// 所以key结点的后继 successorBTNP 肯定位于key结点的右子树中,
			// 且 successorBTNP 没有左孩子( 不然 successorBTNP 的左孩子就是key结点的后继了嘛)
			BTNP tempTempBNTP = tempBNTP->RightNode;

			// key结点的后继
			BTNP successorBTNP = NULL;

			// key结点的后继的父结点
			BTNP successorParentBTNP = NULL;

			while (tempTempBNTP)
			{
				successorBTNP = tempTempBNTP;
				if (tempTempBNTP && tempTempBNTP->LeftNode && tempTempBNTP->LeftNode->LeftNode == NULL)
				{
					successorParentBTNP = tempTempBNTP;
				}
				tempTempBNTP = tempTempBNTP->LeftNode;
			}

			// 情况4又分两种情况, 如下 : 
			// 第一种情况 A 是 successorBTNP 是 key 的右孩子;
			// 第二种情况 B 是 successorBTNP 在 key 的右子树中, 但并不是 successorBTNP 本身并不是 key 的右孩子

			// 情况 A : 
			if (successorBTNP == tempBNTP->RightNode)
			{
				// 用 key 结点的后继 successorBTNP 来替代 key 结点
				if (isLeft)
				{
					parentBTNP->LeftNode = successorBTNP;
				}
				else
				{
					parentBTNP->RightNode = successorBTNP;
				}
			}
			// 情况 B :
			else
			{
				// 如果 successorBTNP 有右子树, 则用 successorBTNP 的右子树 代替 原来 successorBTNP 的位置.
				if (successorBTNP->RightNode)
				{
					successorParentBTNP->LeftNode = successorBTNP->RightNode;
				}

				// 用 key 结点的后继 successorBTNP 的data来替换 key 结点的data
				char * tempChar = new char;
				*tempChar = *(char *)successorBTNP->data;
				tempBNTP->data = tempChar;
				tempBNTP = successorBTNP;

				// 下面注释的这块代码可以用上面这两4行代替
				// if (isLeft)
				// {
				// 	parentBTNP->LeftNode = successorBTNP;
				// 	successorBTNP->RightNode = tempBNTP->RightNode;
				// 	successorBTNP->LeftNode = tempBNTP->LeftNode;
				// }
				// else
				// {
				// 	parentBTNP->RightNode = successorBTNP;
				// 	successorBTNP->RightNode = tempBNTP->RightNode;
				// 	successorBTNP->LeftNode = tempBNTP->LeftNode;
				// }
			}

		}

		// 记得释放 key 结点
		delete (char *)tempBNTP->data;
		tempBNTP->data = NULL;

		delete tempBNTP;
		tempBNTP = NULL;
	}
}

二叉搜索树之找最低公共祖先

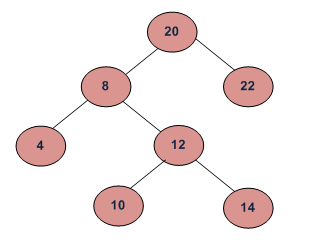
给定二叉搜索树（BST）中两节点，找出他们的最低公共祖先(LeastCommonAncestors, 简称LCA)。

例如对于本文第一张图的LCA为 :

  • LCA(4， 14)=8;
  • LCA(8， 10)=8.

思路:

利用BST的性质, 假设n1,n2都在BST中，并且n1 < n2。则有 :

  • 在遍历过程中，遇到的第一个值介于n1和n2之间的节点n，也即n1 =< n <= n2, 就是n1和n2的LCA。
  • 在遍历过程中，如果节点的值比n1和n2都大，那么LCA在节点的左子树。
  • 在遍历过程中，如果节点的值比n1和n2都小，那么LCA在节点的右子树。
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
void LeastCommonAncestorsBinaraySearchTree(BTNP btnp, char key1, char key2)
{
	if (!btnp)
	{
		cout << "the BST is null" << endl;
		return;
	}

	if (key1 == key2)
	{
		cout << "key1 == key2, so this is not a BST" << endl;
		return;
	}

	if (!SearchBinarySearchTree(btnp, key1))
	{
		cout << "key1 not found" << endl;
		return;
	}

	if (!SearchBinarySearchTree(btnp, key2))
	{
		cout << "key2 not found" << endl;
		return;
	}

	while (btnp)
	{
		char curChar = *(char *)(btnp->data);

		if (curChar < key1 && curChar < key2)
		{
			btnp = btnp->RightNode;
		}
		else if (curChar > key1 && curChar > key2)
		{
			btnp = btnp->LeftNode;
		}
		else 
		{
			cout << "LeastCommonAncestors is " << curChar << endl;
			return;
		}
	}
}

参考

<< 算法导论 >>